Joranalogue Orbit 3 Handleiding

Joranalogue Niet gecategoriseerd Orbit 3

Lees hieronder de 📖 handleiding in het Nederlandse voor Joranalogue Orbit 3 (8 pagina's) in de categorie Niet gecategoriseerd. Deze handleiding was nuttig voor 34 personen en werd door 2 gebruikers gemiddeld met 4.5 sterren beoordeeld

Download PDF
Pagina 1/8
ORBIT 3
DOUBLE- SCROLL CHAOS
OSCILLATOR
1
INTRODUC TION
In everyday usage, 'chaos' means 'disorder,
'randomness'. In mathematics however, chaos
has a very specific meaning. Chaos theory deals
with systems exhibiting highly irregular
behaviour, seemingly random yet completely
deterministic.
Applying this to the world of modular synths
opens up a whole new world of modulation
opportunities, introducing a natural irregularity
to otherwise purely ‘mechanical’ music, yet with
much more control and repeatability than just
simple randomness.
In chaos theory, the combination of all possible
oscillations at a specific set of parameters is
known as the ‘attractor’. Orbit 3 incorporates a
modified electronic analogue of the classic
double-scroll attractor. Since it contains some
(bi)stable regions in addition to chaotic ones, this
type is known as a ‘strange’ attractor.
The voltages at each of the module’s outputs can
be thought to describe the orbit of a particle
through 3D space around two equilibrium
points. The positions of these points, as well as
the distribution of the orbits and rate of
movement can all be adjusted under manual or
voltage control.
Not just limited to low frequency modulation
use, Orbit 3 can operate in the audio frequency
domain as well, yielding a variety of waveforms
from its three complementary output pairs.
These span a wide range from near, -sine waves
to white noise. The addition of temperature
compensation and calibrated pitch tracking
makes for an unconventional and lively, yet also
accurate audio oscillator.
And when you need a break from all that musical
chaos, simply connecting any pair of outputs to
the XY inputs of an oscilloscope will surely not
disappoint!
CONTENTS
In the Orbit 3 box, you’ll find:
 Product card, stating serial number and
production batch.
 16- - -to 10 pin Eurorack power cable.
 Mounting hardware: four black M3 x 6 mm
hex screws, four black nylon washers and a
hex key.
 T 3 he Orbit module itself, in a protective
cotton bag.
If any of these items are missing, please contact
your dealer or support@joranalogue.com.
ORBIT 3
DOUBLE- SCROLL CHAOS
OSCILLATOR
2
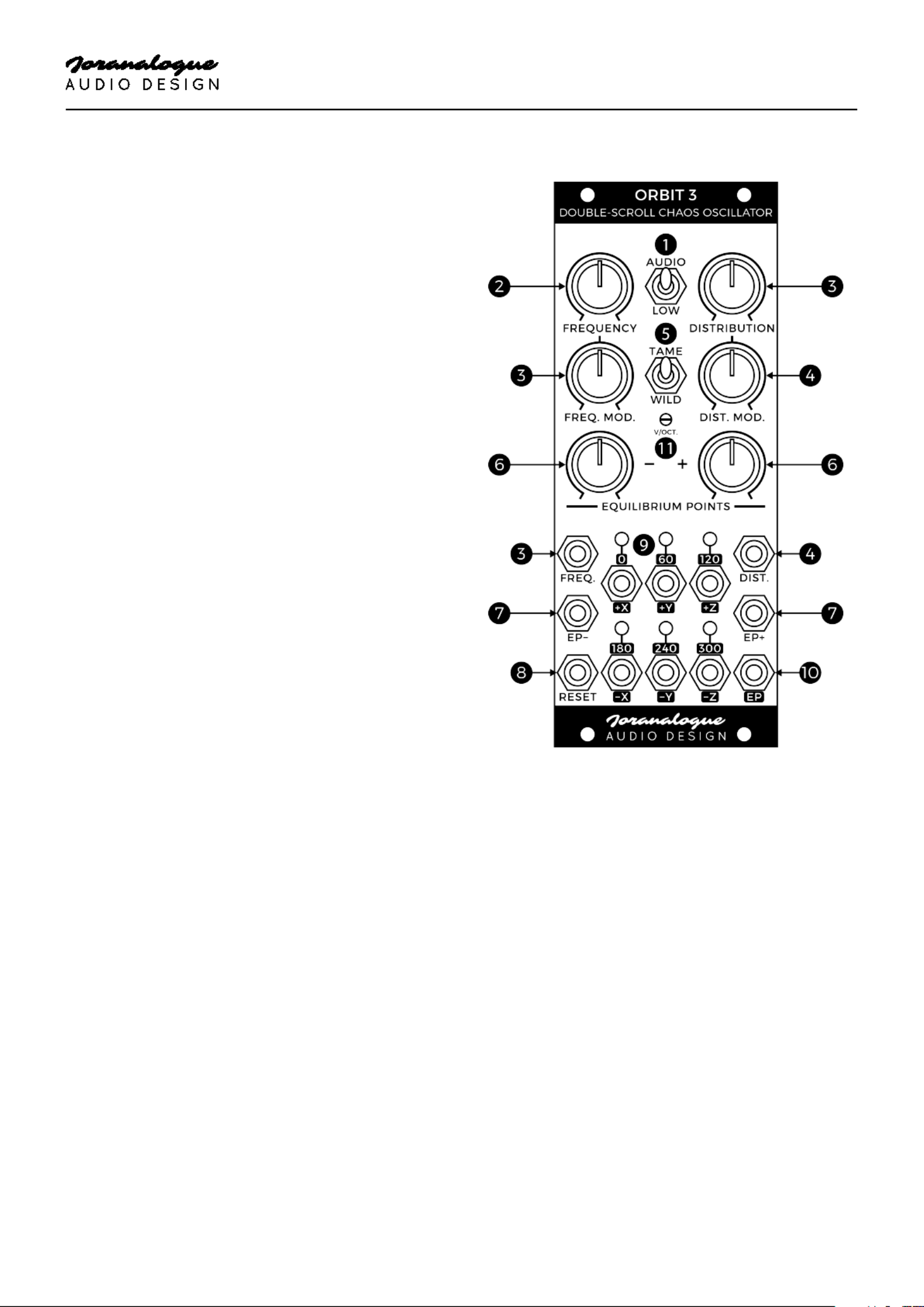
CONTROLS & CONNECTIONS
1 FREQUENCY RANGE SWITCH
This switch determines over which frequency
range Orbit 3 will operate: low frequency (VCLFO)
or audio frequency (VCO).
2 FREQUENCY KNOB
Although it may not always be apparent with
highly irregular chaotic signals, there is a
constant underlying oscillation frequency. This
drives the chaos and sets the rate at which the
signals evolve. It is controlled by this knob, with a
range of 22 Hz to 22 kHz in the audio frequency
mode.
In low frequency mode, the total range is
2 7 .4 mHz (a period of minutes) to 180 Hz, with
1 Hz when the knob is centred.
3 FREQUENCY MODULATION INPUT AND
KNOB
This input is used to modulate the frequency in
an exponential fashion. It includes a polariser
knob to set the modulation depth, with 0 in the
centre, standard +1 volt per octave maximum
and −1 volt per octave minimum in audio mode.
In low mode, the sensitivity is increased to
approximately 0.6 volt per octave.
3 DISTRIBUTION KNOB
The distribution kn ob manipulates Orbit 3’s
internal feedback path to change the nature of
the chaos generated by ‘widening’ the orbits.
When configured with a large distribution, the
oscillator will typically orbit closer to each of the
equilibrium points and spend more time around
it before switching to the other point.
4 DISTRIBUTION MODULATION INPUT AND
KNOB
The distribution parameter can be modulated
using this input, which also includes a polariser
knob. A modulation value of +5 V results in
maximum distribution.
ORBIT 3
DOUBLE- SCROLL CHAOS
OSCILLATOR
3
5 TAME/WILD SWITCH
This switch presents another way to affect the
kind of chaotic signals that are generated. In
mild mode, the orbits take the form of two
distinct scrolls. In wild mode, changing scrolls
becomes much easier, usually resulting in more
irregular orbits.
6 EQUILIBRIUM POINT KNOBS
Rotating either of these knobs clockwise moves
the corresponding equilibrium point further
away from the origin (0 V). As such, these
parameters affect the output amplitudes as well
as the nature of the chaotic signals. Additionally,
setting both points in different positions results
in asymmetric attractors, and changing one
point affects both scrolls.
Any combination of the system parameters may
result in stable oscillation (much like a standard
VCO), bistable operation (cycling between two
different orbits) or full chaos, which is typical of
chaotic systems.
7 EQUILIBRIUM POINT MODULATION INPUTS
Both e quilibrium points can be voltage
modulated using these inputs with 0 V and , +5 V
corresponding to the minimum and maximum
values . for each scroll
8 RESET INPUT
Orbit 3’s core can be instantly by reset a rising
edge on this input, much like the ‘ ’ hard sync on
a conventional oscillator. Depending on the
chaos parameter values, the direction oscillation
may also flip during each reset pulse, leading to
octave down effects at audio frequencies.
This input is uniquely designed to be driven
reliably even from weak, slow, bipolar signals. It
features Schmitt action, with a +2 V low and +3 V
high logic threshold.
9 S CHAO OUTPUTS
These are the outputs from the different stages
inside the chaotic oscillator’s core. They
represent a three- e dimensional orbit on ax s X, Y
and Z . around two adjustable equilibrium points
The output signal of each successive stage will be
phase shifted by 60°, as shown on the front panel,
although the irregular nature of chaos means
this is not always apparent. For patching
convenience, inverted outputs are included as
well, yielding a full set of 6- phase outputs.
The output amplitudes typically range between
3 and 12 Vpp, as determined by the tame/wild
switch and the distribution and scroll position
parameters.
The images on the following page illustrate the
typical figures that arise when projecting the 3D
orbits onto two dimensions, with a variety of
parameter values and in each case using only
two out of six outputs. They are based on true
output . captures of the module in operation

Product specificaties

Merk: Joranalogue
Categorie: Niet gecategoriseerd
Model: Orbit 3

Heb je hulp nodig?

Als je hulp nodig hebt met Joranalogue Orbit 3 stel dan hieronder een vraag en andere gebruikers zullen je antwoorden


Handleiding Niet gecategoriseerd Joranalogue

Joranalogue

Joranalogue Cycle 5 Handleiding

3 Juli 2024
Joranalogue

Joranalogue Contour 1 Handleiding

29 Juni 2023
Joranalogue

Joranalogue Route 4 Handleiding

21 Juni 2023
Joranalogue

Joranalogue Generate 3 Handleiding

20 Juni 2023
Joranalogue

Joranalogue Step 8 Handleiding

19 Juni 2023
Joranalogue

Joranalogue Select 2 Handleiding

19 Juni 2023
Joranalogue

Joranalogue Compare 2 Handleiding

16 Juni 2023
Joranalogue

Joranalogue Orbit 3 Handleiding

15 Juni 2023
Joranalogue

Joranalogue Enhance 2 Handleiding

15 Juni 2023
Joranalogue

Joranalogue Morph 4 Handleiding

14 Juni 2023

Handleiding Niet gecategoriseerd

Nieuwste handleidingen voor Niet gecategoriseerd

Taga Harmony

Taga Harmony TOS-600 v.2 Handleiding

26 November 2024
Trace Elliot

Trace Elliot Pro 2x12 Cabinet Handleiding

26 November 2024
Kramer

Kramer K-Bar Camera Bracket Handleiding

26 November 2024
Kramer

Kramer RK-19 Handleiding

26 November 2024
Kramer

Kramer OSP-MM1 Handleiding

26 November 2024
Kramer

Kramer ASPEN-1616UX Handleiding

26 November 2024
Korg

Korg Pitchstrap Handleiding

26 November 2024
NeoMounts

NeoMounts WL70-450BL11 Handleiding

26 November 2024
Kramer

Kramer CLS-AOCH/60 Handleiding

26 November 2024
Klauke

Klauke EKM 6022ISM Handleiding

26 November 2024